|
|
|
Define the ``information function'' to be
![\begin{displaymath}
I \equiv -\sum_{i=1}^{N} P_i(\epsilon)\ln[P_i(\epsilon)],
\end{displaymath}](i_522.gif) |
(1) |
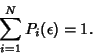 |
(2) |
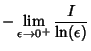 |
|||
![$\displaystyle \lim_{\epsilon\to 0^+} \sum_{i=1}^{N} {P_i(\epsilon)\ln[P_i(\epsilon)]\over\ln(\epsilon)}.$](i_528.gif) |
(3) |
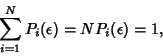 |
(4) |
| (5) |
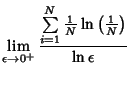 |
|||
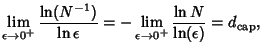 |
(6) |
See also Correlation Exponent
References
Farmer, J. D. ``Chaotic Attractors of an Infinite-dimensional Dynamical System.'' Physica D 4, 366-393,
1982.
Nayfeh, A. H. and Balachandran, B. Applied Nonlinear Dynamics: Analytical, Computational, and Experimental
Methods. New York: Wiley, pp. 545-547, 1995.