|
|
|
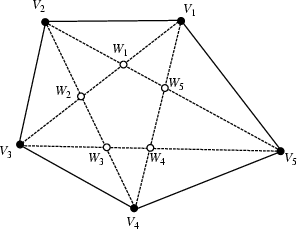
A geometric theorem related to the Pentagram and also called the Pratt-Kasapi Theorem.
References
Chou, S. C. Mechanical Geometry Theorem Proving. Dordrecht, Netherlands: Reidel, 1987.
Grünbaum, B. and Shepard, G. C. ``Ceva, Menelaus, and the Area Principle.'' Math. Mag. 68, 254-268,
1995.
Hoehn, L. ``A Menelaus-Type Theorem for the Pentagram.'' Math. Mag. 68, 254-268, 1995.
Pinkernell, G. M. ``Identities on Point-Line Figures in the Euclidean Plane.'' Math. Mag. 69, 377-383, 1996.