|
|
|
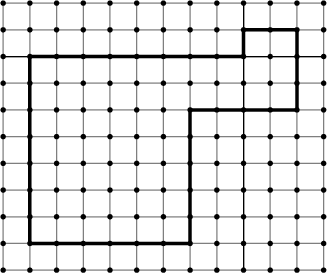
A Plane path on a set of equally spaced Lattice Points, starting at the Origin,
where the first step is one unit to the north or south, the second step is two units to the east or west, the third is three
units to the north or south, etc., and continuing until the Origin is again reached. No crossing or backtracking
is allowed. The simplest golygon is (0, 0), (0, 1), (2, 1), (2, ![]() ), (
), (![]() ,
, ![]() ), (
), (![]() ,
, ![]() ), (
), (![]() ,
, ![]() ), (
), (![]() , 0),
(0, 0).
, 0),
(0, 0).
A golygon can be formed if there exists an Even Integer ![]() such that
such that
| (1) | |||
| (2) |
| (3) |
| (4) |
| (5) |
See also Lattice Path
References
Dudeney, A. K. ``An Odd Journey Along Even Roads Leads to Home in Golygon City.'' Sci. Amer. 263, 118-121,
July 1990.
Sallows, L. C. F. ``New Pathways in Serial Isogons.'' Math. Intell. 14, 55-67, 1992.
Sallows, L.; Gardner, M.; Guy, R. K.; and Knuth, D. ``Serial Isogons of 90 Degrees.'' Math Mag. 64,
315-324, 1991.
Sloane, N. J. A. Sequence
A006718/M3707
in ``An On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.
The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995.
Vardi, I. ``American Science.'' §5.3 in
Computational Recreations in Mathematica. Redwood City, CA: Addison-Wesley,
pp. 90-96, 1991.
|
|
|
© 1996-9 Eric W. Weisstein