A.k.a. Gauss's Theorem. Let 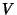 be a region in space with boundary
be a region in space with boundary 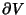 . Then
. Then
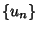 |
(1) |
Let 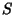 be a region in the plane with boundary
be a region in the plane with boundary 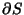 .
.
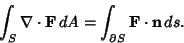 |
(2) |
If the Vector Field 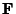 satisfies certain constraints, simplified forms can be used. If
satisfies certain constraints, simplified forms can be used. If
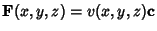 where
where 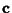 is a constant vector
is a constant vector 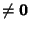 , then
, then
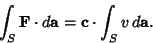 |
(3) |
But
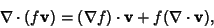 |
(4) |
so
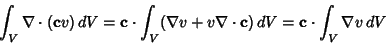 |
(5) |
 |
(6) |
But
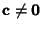 , and
, and
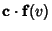 must vary with
must vary with  so that
so that
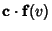 cannot
always equal zero. Therefore,
cannot
always equal zero. Therefore,
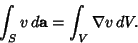 |
(7) |
If
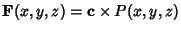 , where
, where 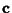 is a constant vector
is a constant vector 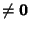 , then
, then
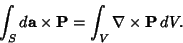 |
(8) |
See also Curl Theorem, Gradient, Green's Theorem
References
Arfken, G. ``Gauss's Theorem.'' §1.11 in
Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 57-61, 1985.
© 1996-9 Eric W. Weisstein
1999-05-24
![]() be a region in space with boundary
be a region in space with boundary ![]() . Then
. Then