Dedekind Eta Function

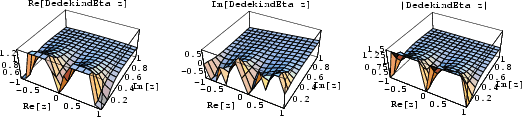
$\begin{figure}\begin{center}\BoxedEPSF{DedekindEtaReIm.epsf scaled 620}\end{center}\end{figure}$

Let

$\begin{displaymath} q=e^{2\pi iz}, \end{displaymath}$

(1)

then the Dedekind eta function is defined over the upper half-plane $H=\{\tau:\Im[\tau]>0\}$ by

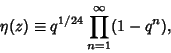$\begin{displaymath} \eta(z)\equiv q^{1/24}\prod_{n=1}^\infty (1-q^{n}), \end{displaymath}$

(2)

which can be written as

$\begin{displaymath} \eta(z)=q^{1/24}\left\{{1+\sum_{n=1}^\infty (-1)^n [q^{n(3n-1)/2}+q^{n(3n+1)/2}]}\right\} \end{displaymath}$

(3)

(Weber 1902, pp. 85 and 112; Atkin and Morain 1993). $\eta$ is a Modular Form. Letting $\zeta_{24}=e^{2\pi i/24}$ be a Root of Unity, $\eta(z)$ satisfies

$\displaystyle \eta(z+1)$	$\textstyle =$	$\displaystyle \zeta_{24}\eta(z)$	(4)
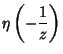$\displaystyle \eta\left({-{1\over z}}\right)$	$\textstyle =$	$\displaystyle \sqrt{-iz}\,\eta(z)$	(5)

(Weber 1902, p. 113; Atkin and Morain 1993).

References

Atkin, A. O. L. and Morain, F. ``Elliptic Curves and Primality Proving.'' Math. Comput. 61, 29-68, 1993.

Weber, H. Lehrbuch der Algebra, Vols. I-II. New York: Chelsea, 1902.