|
|
|
The ![]() phase factor in some definitions of the Spherical Harmonics and associated
Legendre Polynomials. Using the Condon-Shortley convention gives
phase factor in some definitions of the Spherical Harmonics and associated
Legendre Polynomials. Using the Condon-Shortley convention gives
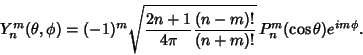
See also Legendre Polynomial, Spherical Harmonic
References
Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 682 and 692, 1985.
Condon, E. U. and Shortley, G. The Theory of Atomic Spectra. Cambridge, England: Cambridge University Press, 1951.
Shore, B. W. and Menzel, D. H. Principles of Atomic Spectra. New York: Wiley, p. 158, 1968.