|
|
|
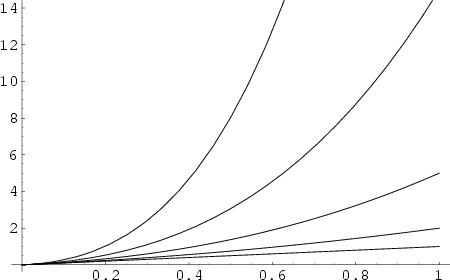
Two different Generating Functions for the Bell polynomials for ![]() are given by
are given by
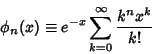
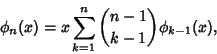
The Bell polynomials are defined such that ![]() , where
, where ![]() is a Bell Number. The first few Bell
polynomials are
is a Bell Number. The first few Bell
polynomials are
See also Bell Number
References
Bell, E. T. ``Exponential Polynomials.'' Ann. Math. 35, 258-277, 1934.