An antisymmetric tensor is defined as a Tensor for which
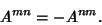 |
(1) |
Any Tensor can be written as a sum of Symmetric and antisymmetric parts as
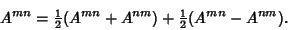 |
(2) |
The antisymmetric part is sometimes denoted using the special notation
![\begin{displaymath}
A^{[ab]} = {\textstyle{1\over 2}}(A^{ab}-A^{ba}).
\end{displaymath}](a_1107.gif) |
(3) |
For a general Tensor,
![\begin{displaymath}
A^{[a_1 \cdots a_n]} \equiv {1\over n!} \epsilon_{a_1 \cdots a_n} \sum_{\rm permutations} A^{a_1 \cdots a_n},
\end{displaymath}](a_1108.gif) |
(4) |
where
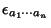 is the Levi-Civita Symbol, a.k.a. the Permutation Symbol.
is the Levi-Civita Symbol, a.k.a. the Permutation Symbol.
See also Symmetric Tensor
© 1996-9 Eric W. Weisstein
1999-05-25
![\begin{displaymath}
A^{[a_1 \cdots a_n]} \equiv {1\over n!} \epsilon_{a_1 \cdots a_n} \sum_{\rm permutations} A^{a_1 \cdots a_n},
\end{displaymath}](a_1108.gif)