|
|
|
A set of identities involving ![]() -D visible lattice points was discovered by Campbell (1994). Examples include
-D visible lattice points was discovered by Campbell (1994). Examples include
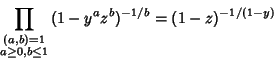
![\begin{displaymath}
\prod_{\scriptstyle (a,b,c)=1\atop\scriptstyle a,b\geq 0, c\leq 1} (1-x^ay^bz^c)^{-1/c}=(1-z)^{-1/[(1-x)(1-y)]}
\end{displaymath}](v_635.gif)
References
Campbell, G. B. ``Infinite Products Over Visible Lattice Points.'' Internat. J. Math. Math. Sci. 17, 637-654, 1994.
Campbell, G. B. ``Visible Point Vector Identities.''
http://www.geocities.com/CapeCanaveral/Launchpad/9416/vpv.html.