|
|
|
|
An Archimedean Solid whose Dual Polyhedron is the Rhombic Triacontahedron. It is one of the two convex
Quasiregular Polyhedra and has Schläfli Symbol
![]() . It is also Uniform Polyhedron
. It is also Uniform Polyhedron ![]() and has Wythoff Symbol
and has Wythoff Symbol ![]() . Its
faces are
. Its
faces are
![]() . The Vertices of an icosidodecahedron of Edge length
. The Vertices of an icosidodecahedron of Edge length ![]() are
are ![]() ,
, ![]() ,
, ![]() ,
,
![]() ,
,
![]() ,
,
![]() . The 30 Vertices of an
Octahedron 5-Compound form an icosidodecahedron (Ball and Coxeter 1987). Faceted versions include the Small
Icosihemidodecahedron and Small Dodecahemidodecahedron.
. The 30 Vertices of an
Octahedron 5-Compound form an icosidodecahedron (Ball and Coxeter 1987). Faceted versions include the Small
Icosihemidodecahedron and Small Dodecahemidodecahedron.
The faces of the icosidodecahedron consist of 20 triangles and 12 pentagons. Furthermore, its 60 edges are bisected perpendicularly by those of the reciprocal Rhombic Triacontahedron (Ball and Coxeter 1987).
The Inradius, Midradius, and Circumradius for unit edge length are
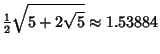 |
|||
See also Archimedean Solid, Great Icosidodecahedron, Quasiregular Polyhedron, Small Icosihemidodecahedron, Small Dodecahemidodecahedron
References
Ball, W. W. R. and Coxeter, H. S. M. Mathematical Recreations and Essays, 13th ed. New York:
Dover, p. 137, 1987.
Wenninger, M. J. Polyhedron Models. Cambridge, England: Cambridge University Press, p. 73, 1989.