|
|
|
N.B. A detailed on-line essay by S. Finch was the starting point for this entry.
Let ![]() be the number of 1s in the Binary expression of
be the number of 1s in the Binary expression of ![]() . Then the number of Odd Binomial
Coefficients
. Then the number of Odd Binomial
Coefficients ![]() where
where ![]() is
is ![]() (Glaisher 1899, Fine 1947). The
number of Odd elements in the first
(Glaisher 1899, Fine 1947). The
number of Odd elements in the first ![]() rows of Pascal's Triangle is
rows of Pascal's Triangle is
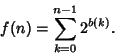 |
(1) |
| (2) |
| (3) |
| (4) |
References
Finch, S. ``Favorite Mathematical Constants.'' http://www.mathsoft.com/asolve/constant/stlrsky/stlrsky.html
Fine, N. J. ``Binomial Coefficients Modulo a Prime.'' Amer. Math. Monthly 54, 589-592, 1947.
Wolfram, S. ``Geometry of Binomial Coefficients.'' Amer. Math. Monthly 91, 566-571, 1984.