For 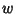 a Differential k-Form with compact support on an oriented
a Differential k-Form with compact support on an oriented 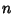 -dimensional
Manifold
-dimensional
Manifold 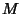 ,
,
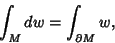 |
(1) |
where 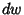 is the Exterior Derivative of the differential form
is the Exterior Derivative of the differential form 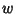 . This connects to the ``standard''
Gradient, Curl, and Divergence Theorems
by the following relations. If
. This connects to the ``standard''
Gradient, Curl, and Divergence Theorems
by the following relations. If 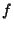 is a function on
is a function on 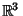 ,
,
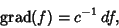 |
(2) |
where
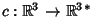 (the dual space) is the duality isomorphism between a Vector Space
and its dual, given by the Euclidean Inner Product on
(the dual space) is the duality isomorphism between a Vector Space
and its dual, given by the Euclidean Inner Product on 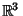 . If
. If 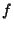 is a Vector Field on a
is a Vector Field on a
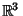 ,
,
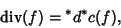 |
(3) |
where 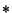 is the Hodge Star operator. If
is the Hodge Star operator. If 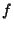 is a Vector Field on
is a Vector Field on 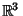 ,
,
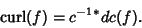 |
(4) |
With these three identities in mind, the above Stokes' theorem in the three instances is transformed into the
Gradient, Curl, and Divergence Theorems
respectively as follows. If 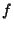 is a function on
is a function on 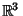 and
and 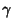 is a curve in
is a curve in 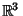 , then
, then
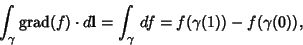 |
(5) |
which is the Gradient Theorem. If
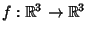 is a Vector Field and
is a Vector Field and 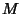 an embedded compact
3-manifold with boundary in
an embedded compact
3-manifold with boundary in 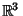 , then
, then
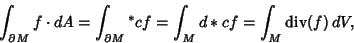 |
(6) |
which is the Divergence Theorem. If 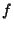 is a Vector Field and
is a Vector Field and 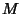 is an oriented, embedded, compact
2-Manifold with boundary in
is an oriented, embedded, compact
2-Manifold with boundary in 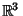 , then
, then
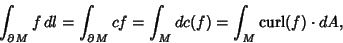 |
(7) |
which is the Curl Theorem.
Physicists generally refer to the Curl Theorem
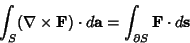 |
(8) |
as Stokes' theorem.
See also Curl Theorem, Divergence Theorem, Gradient Theorem
© 1996-9 Eric W. Weisstein
1999-05-26
![]() a Differential k-Form with compact support on an oriented
a Differential k-Form with compact support on an oriented ![]() -dimensional
Manifold
-dimensional
Manifold ![]() ,
,
![]() is a function on
is a function on ![]() and
and ![]() is a curve in
is a curve in ![]() , then
, then