Given a set 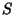 with a subset
with a subset 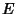 , the complement of
, the complement of 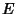 is defined as
is defined as
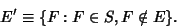 |
(1) |
If 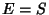 , then
, then
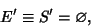 |
(2) |
where 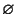 is the Empty Set. Given a single Set, the second Probability Axiom gives
is the Empty Set. Given a single Set, the second Probability Axiom gives
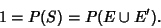 |
(3) |
Using the fact that
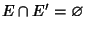 ,
,
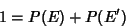 |
(4) |
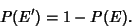 |
(5) |
This demonstrates that
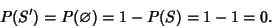 |
(6) |
Given two Sets,
© 1996-9 Eric W. Weisstein
1999-05-26
![]() with a subset
with a subset ![]() , the complement of
, the complement of ![]() is defined as
is defined as