Circle Packing

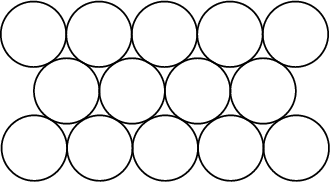
The densest packing of circles in the Plane is the hexagonal lattice of the bee's honeycomb (illustrated above), which has a Packing Density of

$$\eta={\pi\over 2\sqrt{3}}=0.9068996821\ldots.$$

Gauß proved that the hexagonal lattice is the densest plane lattice packing, and in 1940, L. Fejes Tóth proved that the hexagonal lattice is indeed the densest of all possible plane packings.

Solutions for the smallest diameter Circles into which $n$ Unit Circles can be packed have been proved optimal for $n=1$ through 10 (Kravitz 1967). The best known results are summarized in the following table.

$n$	$d$ exact	$d$ approx.
1	1	1.00000
2	2	2.00000
3	$1+{\textstyle{2\over 3}}\sqrt{3}$	2.15470...
4	$1+\sqrt{2}$	2.41421...
5	$1+\sqrt{2(1+1/\sqrt{5})}$	2.70130...
6	3	3.00000
7	3	3.00000
8	$1+\csc(\pi/7)$	3.30476...
9	$1+\sqrt{2(2+\sqrt{2}\,)}$	3.61312...
10		3.82...
11
12		4.02...

For Circle packing inside a Square, proofs are known only for $n=1$ to 9.

$n$	$d$ exact	$d$ approx.
1	1	1.000
2		0.58...
3		0.500...
4	${\textstyle{1\over 2}}$	0.500
5		0.41...
6		0.37...
7		0.348...
8		0.341...
9	${\textstyle{1\over 3}}$	0.333...
10		0.148204...

The smallest Square into which two Unit Circles, one of which is split into two pieces by a chord, can be packed is not known (Goldberg 1968, Ogilvy 1990).

See also Hypersphere Packing, Malfatti's Right Triangle Problem, Mergelyan-Wesler Theorem, Sphere Packing

References

Conway, J. H. and Sloane, N. J. A. Sphere Packings, Lattices, and Groups, 2nd ed. New York: Springer-Verlag, 1992.

Eppstein, D. ``Covering and Packing.'' http://www.ics.uci.edu/~eppstein/junkyard/cover.html.

Folkman, J. H. and Graham, R. ``A Packing Inequality for Compact Convex Subsets of the Plane.'' Canad. Math. Bull. 12, 745-752, 1969.

Gardner, M. ``Mathematical Games: The Diverse Pleasures of Circles that Are Tangent to One Another.'' Sci. Amer. 240, 18-28, Jan. 1979.

Gardner, M. ``Tangent Circles.'' Ch. 10 in Fractal Music, Hypercards, and More Mathematical Recreations from Scientific American Magazine. New York: W. H. Freeman, 1992.

Goldberg, M. ``Problem E1924.'' Amer. Math. Monthly 75, 195, 1968.

Goldberg, M. ``The Packing of Equal Circles in a Square.'' Math. Mag. 43, 24-30, 1970.

Goldberg, M. ``Packing of 14, 16, 17, and 20 Circles in a Circle.'' Math. Mag. 44, 134-139, 1971.

Graham, R. L. and Luboachevsky, B. D. ``Repeated Patterns of Dense Packings of Equal Disks in a Square.'' Electronic J. Combinatorics 3, R16 1-17, 1996. http://www.combinatorics.org/Volume_3/volume3.html#R16.

Kravitz, S. ``Packing Cylinders into Cylindrical Containers.'' Math. Mag. 40, 65-70, 1967.

McCaughan, F. ``Circle Packings.'' http://www.pmms.cam.ac.uk/~gjm11/cpacking/info.html.

Molland, M. and Payan, Charles. ``A Better Packing of Ten Equal Circles in a Square.'' Discrete Math. 84, 303-305, 1990.

Ogilvy, C. S. Excursions in Geometry. New York: Dover, p. 145, 1990.

Reis, G. E. ``Dense Packing of Equal Circle within a Circle.'' Math. Mag. 48, 33-37, 1975.

Schaer, J. ``The Densest Packing of Nine Circles in a Square.'' Can. Math. Bul. 8, 273-277, 1965.

Schaer, J. ``The Densest Packing of Ten Equal Circles in a Square.'' Math. Mag. 44, 139-140, 1971.

Valette, G. ``A Better Packing of Ten Equal Circles in a Square.'' Discrete Math. 76, 57-59, 1989.